Teachers: Anonymous
Source: Internet
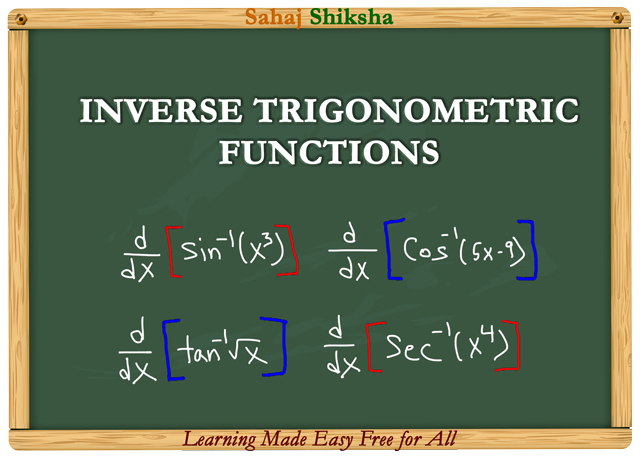
In class 11 maths, students have already learnt trigonometric function. The inverse trigonometric function is the inverse of a trigonometric function.
In this chapter of maths, students will learn concepts related to the definition of inverse trigonometric functions, domain, range, and principal value, graph, and properties of inverse trigonometric functions.
Students have already learnt that trigonometric functions are not one to one and onto in their natural ranges. All these questions & solutions are explained in step-by-step method for class 12 students. These are important while practice the problems and in depth understanding of this section.
ক্লাস 11 গণিতে, শিক্ষার্থীরা ইতিমধ্যে ত্রিকোণমিতিক অপেক্ষকসমূহ শিখেছে। বিপরীত ত্রিকোণমিতিক অপেক্ষকসমূহ হল একটি ত্রিকোণমিতিক অপেক্ষকসমূহের বিপরীত।
গণিতের এই অধ্যায়ে, শিক্ষার্থীরা বিপরীত ত্রিকোণমিতিক অপেক্ষকসমূহ সংজ্ঞা সম্পর্কিত ধারণাগুলি শিখবে। শিক্ষার্থীরা ইতিমধ্যেই শিখেছে যে ত্রিকোণমিতিক অপেক্ষকসমূহগুলি তাদের প্রাকৃতিক সীমার মধ্যে এক থেকে এক নয়।
এই সমস্ত প্রশ্ন এবং সমাধান 12 শ্রেনীর শিক্ষার্থীদের জন্য ধাপে ধাপে পদ্ধতিতে ব্যাখ্যা করা হয়েছে। এই বিভাগের সমস্যা এবং গভীরভাবে বোঝার অনুশীলন করার সময় এগুলি গুরুত্বপূর্ণI
Question:1
Question:2
Question:3
Question:4
Find the principal values of
Answer:
Let us assume that ,
then we have or
and as we know that the principal value of is
.
Hence the only principal value of when
.
Question:5
Find the principal values of
Answer:
Let us assume that then,
Easily we have or
we can write it as
as we know that the range of the principal values of is
.
Hence lies in the range it is a principal solution.
Question:6
Find the principal values of
Answer:
Given so we can assume it to be equal to ‘z’
,
or
And as we know the range of principal values of from
.
As only one value z = lies hence we have only one principal value that is
.
Question:7
Find the principal values of
Answer:
Let us assume that then,
we can also write it as; .Or
and the principal values lies between .
Hence we get only one principal value of i.e.,
.
Question:8
Find the principal values of
Answer:
Let us assume that ,
then we can write in other way or
.
Hence when we have
and the range of principal values of lies in
.
Then the principal value of is
Question:9
Find the principal values of
Answer:
Let us assume ;
Then we have or
,
.
And we know the range of principal values of is
.
So, the only principal value which satisfies is
.
Question:10
Find the principal values of
Answer:
Let us assume the value of ,
then we have or
and the range of the principal values of lies between
hence the principal value of is
.
Question:11
Find the principal values of
Answer:
To find the values first we declare each term to some constant ,
So we have or
Therefore &
So, we have .
Therefore ,
,
So we have or
Therefore
Hence we can calculate the sum
Question:12
Find the principal values of
Answer:
Here we have
let us assume that the value of
then we have to find out the value of x +2y.
Calculation of x
Hence .
Calculation of y
.
Hence .
The required sum will be =
Question:13
Prove the following
Answer:
Given to prove where
.
Take or
Take R.H.S value
= =
=
=
= L.H.S
Question:14
Prove that
Answer:
Given to prove .
Take or
;
Then we have R.H.S.
=
=
=
=
= L.H.S
Hence Proved.
Question:15
Prove that
Answer:
Given to prove
We have L.H.S
= R.H.S
Hence proved.
Question:16
Prove that
Answer:
Given to prove
Then taking L.H.S.
We have
= R.H.S.
Hence proved.
Question:17
Write the following functions in the simplest form
Answer:
We have
Take
is the simplified form.
Question:18
Write the simplest form of function or
=
=
Question:19
Write the following functions in the simplest form
Answer:
Given that
We have in inside the root the term :
Put and
,
Then we have,
Hence the simplest form is
![]()